
Then plot each point ( x, x 2) on the coordinate plane and connect the dots with a smooth curve. In other words, at each x-value, plug into the function to determine the corresponding y-value.įor example, to graph f( x) = x 2, evaluate the function at a few points. The graph of a function f is the set of all points ( x, y) that satisfy y = f( x). Here’s a good refresher for domain and range. Then more complicated functions may be analyzed using the properties of scaling and translations.įor example, we can deduce that the range of f( x) = ( x + 3) 2 – 7 is (-7, ∞) because f is just a translation of the graph of x 2 by 7 units down (and 3 units left, which doesn’t affect the range). It helps to memorize the domains and ranges of simple functions first. The range of a function is the set of all output values ( y-values) for the function.įinding the range is quite a bit more challenging than finding domain in general. We find that x = 5 and x = -2 must be excluded. On the other hand, the domain of a rational function such as excludes any x-values for which the denominator evaluates to zero. Any real number at all can serve as input for this function. Unless further information is given, we look for the natural domain, which is the largest set of x-values that makes sense for the function.įor example, the domain of f( x) = x 2 is the set of all real numbers, or (-∞, ∞) in interval notation. The domain of a function f( x) is the set of all input values ( x-values) for the function. Understanding how compositions work helps you to make sense of the Chain Rule for derivatives and the Substitution Rule for integrals.
The last example in the list illustrates a composition of two functions, f and g.
#Limits calculus examples how to
Continuing with our example, f( x) = x 2, let’s see how to evaluate f at various inputs. Evaluating FunctionsĮvaluating a function on a given input is often called plugging in. For example, the squaring function f( x) = x 2 has input x and output y = x 2. Generally a function in calculus is defined by an expression that shows how to calculate the output from any given input. In principle, the input and output could be anything: numbers, vectors, symbols, even other functions! Typically in calculus the input and output are real numbers.Ī function F from X to Y. We use the notation y = f( x) to indicate that x is the input, y is the output, and f is the name of the function.

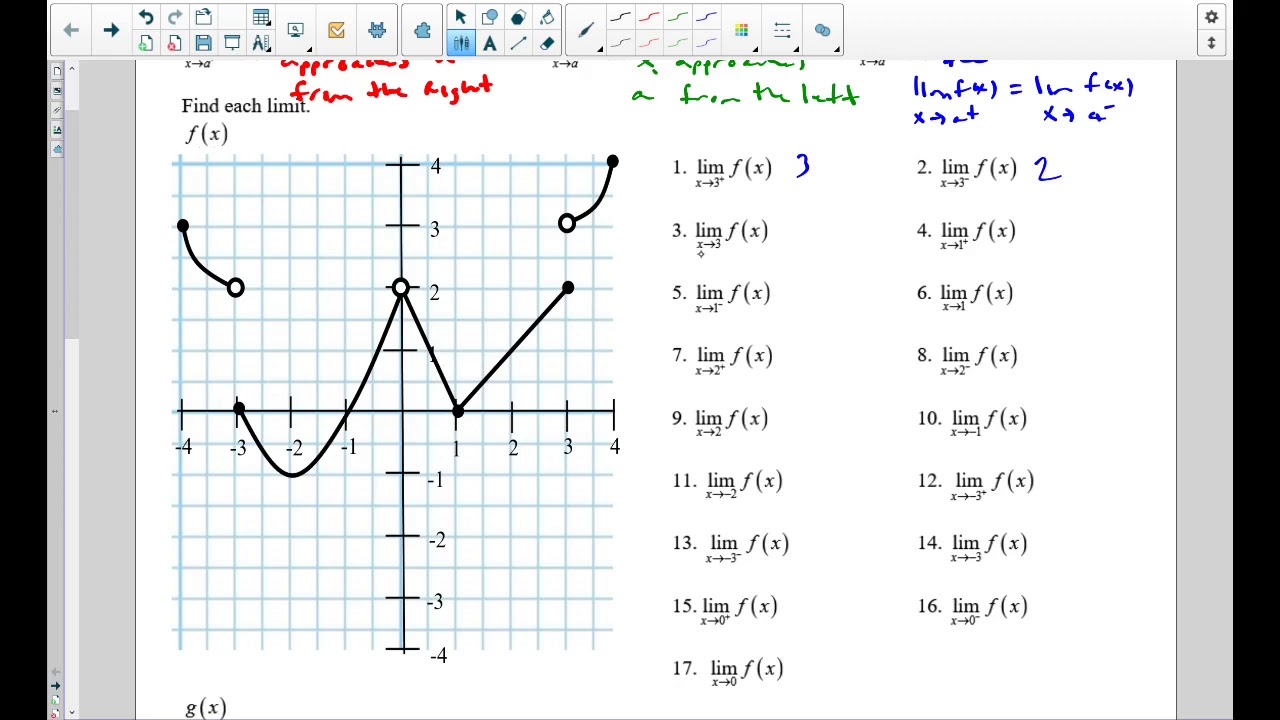
Predicting and approximating the value of a certain set of quantities and even functions is an important goal of calculus. Limits are the foundation of calculus – differential and integral calculus. Limits Calculus – Definition, Properties, and Graphs


 0 kommentar(er)
0 kommentar(er)
